Show Mobile Notice

1. Absolute Min And Max Calc
2. Absolute Minimum Finder
3. Finding Maximum And Minimum Values
4. Absolute Minimum

Absolute minimum definition: 1. The lowest value across a whole domain (= range) of a function: 2. The lowest value across a. Cambridge Dictionary +Plus.

Show All NotesHide All Notes

The absolute minimum - Find potential answers to this crossword clue at crosswordnexus.com. This calculus video tutorial explains how to find the absolute maximum and minimum values of a function on a closed interval. Tto find the absolute extrema.

Section 4-4 : Finding Absolute Extrema

It’s now time to see our first major application of derivatives in this chapter. Indesign free. download full version. Given a continuous function, (fleft( x right)), on an interval (left[ {a,b} right]) we want to determine the absolute extrema of the function. To do this we will need many of the ideas that we looked at in the previous section.

First, since we have a closed interval (i.e. and interval that includes the endpoints) and we are assuming that the function is continuous the Extreme Value Theorem tells us that we can in fact do this. This is a good thing of course. We don’t want to be trying to find something that may not exist.

Next, we saw in the previous section that absolute extrema can occur at endpoints or at relative extrema. Also, from the previous section that we know that the list of critical points is also a list of all possible relative extrema. So, the endpoints along with the list of all critical points will in fact be a list of all possible absolute extrema.

Now we just need to recall that the absolute extrema are nothing more than the largest and smallest values that a function will take so all that we really need to do is get a list of possible absolute extrema, plug these points into our function and then identify the largest and smallest values.

Here is the procedure for finding absolute extrema.

Finding Absolute Extrema of (fleft( x right)) on (left[ {a,b} right])

0. Verify that the function is continuous on the interval (left[ {a,b} right]).
   
1. Find all critical points of (fleft( x right)) that are in the interval (left[ {a,b} right]). This makes sense if you think about it. Since we are only interested in what the function is doing in this interval we don’t care about critical points that fall outside the interval.
   
2. Evaluate the function at the critical points found in step 1 and the end points.
   
3. Identify the absolute extrema.

There really isn’t a whole lot to this procedure. We called the first step in the process step 0, mostly because all of the functions that we’re going to look at here are going to be continuous, but it is something that we do need to be careful with. This process will only work if we have a function that is continuous on the given interval. The most labor intensive step of this process is the second step (step 1) where we find the critical points. It is also important to note that all we want are the critical points that are in the interval.

Let’s do some examples.

Example 1 Determine the absolute extrema for the following function and interval. [gleft( t right) = 2{t^3} + 3{t^2} - 12t + 4hspace{0.25in}{mbox{on}}hspace{0.25in}left[ { - 4,2} right]]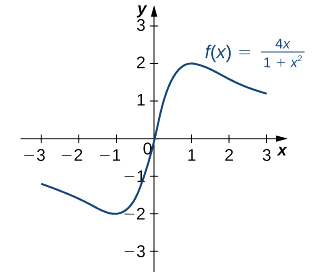 Show Solution

All we really need to do here is follow the procedure given above. So, first notice that this is a polynomial and so is continuous everywhere and therefore is continuous on the given interval.

Now, we need to get the derivative so that we can find the critical points of the function.

[g'left( t right) = 6{t^2} + 6t - 12 = 6left( {t + 2} right)left( {t - 1} right)]

It looks like we’ll have two critical points, (t = - 2) and (t = 1). Note that we actually want something more than just the critical points. We only want the critical points of the function that lie in the interval in question. Both of these do fall in the interval as so we will use both of them. That may seem like a silly thing to mention at this point, but it is often forgotten, usually when it becomes important, and so we will mention it at every opportunity to make sure it’s not forgotten.

Now we evaluate the function at the critical points and the end points of the interval.

[begin{align*}gleft( { - 2} right) & = 24hspace{1.0in} & gleft( 1 right) & = - 3 gleft( { - 4} right) &= - 28hspace{1.0in} & gleft( 2 right) & = 8end{align*}]

Absolute extrema are the largest and smallest the function will ever be and these four points represent the only places in the interval where the absolute extrema can occur. So, from this list we see that the absolute maximum of (gleft( t right)) is 24 and it occurs at (t = - 2) (a critical point) and the absolute minimum of (gleft( t right)) is -28 which occurs at (t = - 4) (an endpoint).

In this example we saw that absolute extrema can and will occur at both endpoints and critical points. One of the biggest mistakes that students make with these problems is to forget to check the endpoints of the interval.

Example 2 Determine the absolute extrema for the following function and interval. [gleft( t right) = 2{t^3} + 3{t^2} - 12t + 4hspace{0.25in}{mbox{on}}hspace{0.25in}left[ {0,2} right]] Show Solution

Note that this problem is almost identical to the first problem. The only difference is the interval that we’re working on. This small change will completely change our answer however. With this change we have excluded both of the answers from the first example.

The first step is to again find the critical points. From the first example we know these are (t = - 2) and (t = 1). At this point it’s important to recall that we only want the critical points that actually fall in the interval in question. This means that we only want (t = 1) since (t = - 2) falls outside the interval.

Now evaluate the function at the single critical point in the interval and the two endpoints.

[gleft( 1 right) = - 3hspace{0.5in}gleft( 0 right) = 4hspace{0.5in}gleft( 2 right) = 8]

From this list of values we see that the absolute maximum is 8 and will occur at (t = 2) and the absolute minimum is -3 which occurs at (t = 1).

As we saw in this example a simple change in the interval can completely change the answer. It also has shown us that we do need to be careful to exclude critical points that aren’t in the interval. Had we forgotten this and included (t = - 2) we would have gotten the wrong absolute maximum!

This is the other big mistakes that students make in these problems. All too often they forget to exclude critical points that aren’t in the interval. If your instructor is anything like me this will mean that you will get the wrong answer. It’s not too hard to make sure that a critical point outside of the interval is larger or smaller than any of the points in the interval.

Example 3 Suppose that the population (in thousands) of a certain kind of insect after (t) months is given by the following formula. [Pleft( t right) = 3,t + sin left( {4t} right) + 100]

Determine the minimum and maximum population in the first 4 months.

Show Solution

The question that we’re really asking is to find the absolute extrema of (Pleft( t right)) on the interval (left[ {0,4} right]). Since this function is continuous everywhere we know we can do this.

Let’s start with the derivative.

[P'left( t right) = 3 + 4cos left( {4t} right)]

We need the critical points of the function. The derivative exists everywhere so there are no critical points from that. So, all we need to do is determine where the derivative is zero.

[begin{align*}3 + 4cos left( {4t} right) & = 0 cos left( {4t} right) & = - frac{3}{4}end{align*}]

The solutions to this are,

[begin{array}{*{20}{c}}{4t = 2.4189 + 2pi n,n = 0, pm 1, pm 2, ldots }{4t = 3.8643 + 2pi n,n = 0, pm 1, pm 2, ldots }end{array}hspace{0.25in} Rightarrow hspace{0.25in}begin{array}{*{20}{c}}{t = 0.6047 + displaystyle frac{{pi n}}{2},n = 0, pm 1, pm 2, ldots }{t = 0.9661 + displaystyle frac{{pi n}}{2},n = 0, pm 1, pm 2, ldots }end{array}]

So, these are all the critical points. We need to determine the ones that fall in the interval (left[ {0,4} right]). There’s nothing to do except plug some (n)’s into the formulas until we get all of them.

(n = 0) :

[t = 0.6047hspace{1.0in}t = 0.9661]

We’ll need both of these critical points.

(n = 1)

[t = 0.6047 + frac{pi }{2} = 2.1755hspace{1.0in}t = 0.9661 + frac{pi }{2} = 2.5369]

We’ll need these.

(n = 2)

[t = 0.6047 + pi = 3.7463hspace{1.0in}t = 0.9661 + pi = 4.1077]

In this case we only need the first one since the second is out of the interval.

There are five critical points that are in the interval. They are,

[0.6047,0.9661,2.1755,2.5369,3.7463]

Finally, to determine the absolute minimum and maximum population we only need to plug these values into the function as well as the two end points. Here are the function evaluations.

[begin{align*}Pleft( 0 right) & = 100.0hspace{1.0in} & Pleft( 4 right) & = 111.7121 Pleft( {0.6047} right) & = 102.4756hspace{1.0in} & Pleft( {0.9661} right) & = 102.2368 Pleft( {2.1755} right) & = 107.1880hspace{1.0in} & Pleft( {2.5369} right) & = 106.9492 Pleft( {3.7463} right) & = 111.9004 & & end{align*}]

From these evaluations it appears that the minimum population is 100,000 (remember that (P) is in thousands…) which occurs at (t = 0) and the maximum population is 111,900 which occurs at (t = 3.7463).

Make sure that you can correctly solve trig equations. If we had forgotten the (2pi n) we would have missed the last three critical points in the interval and hence gotten the wrong answer since the maximum population was at the final critical point.

Also, note that we do really need to be very careful with rounding answers here. If we’d rounded to the nearest integer, for instance, it would appear that the maximum population would have occurred at two different locations instead of only one.

Example 4 Suppose that the amount of money in a bank account after (t) years is given by, [Aleft( t right) = 2000 - 10t{{bf{e}}^{5 - frac{{{t^2}}}{8}}}]

Determine the minimum and maximum amount of money in the account during the first 10 years that it is open.

Show Solution

Here we are really asking for the absolute extrema of (Aleft( t right)) on the interval (left[ {0,10} right]). As with the previous examples this function is continuous everywhere and so we know that this can be done.

We’ll first need the derivative so we can find the critical points.

[begin{align*}A'left( t right) & = - 10{{bf{e}}^{5 - frac{{{t^2}}}{8}}} - 10t{{bf{e}}^{5 - frac{{{t^2}}}{8}}}left( { - frac{t}{4}} right) & = 10{{bf{e}}^{5 - frac{{{t^2}}}{8}}}left( { - 1 + frac{{{t^2}}}{4}} right)end{align*}]

The derivative exists everywhere and the exponential is never zero. Therefore, the derivative will only be zero where,

[ - 1 + frac{{{t^2}}}{4} = 0hspace{0.25in}, Rightarrow hspace{0.25in},{t^2} = 4hspace{0.5in} Rightarrow hspace{0.25in},t = pm 2]

We’ve got two critical points, however only (t = 2) is actually in the interval so that is only critical point that we’ll use.

Let’s now evaluate the function at the lone critical point and the end points of the interval. Here are those function evaluations.

[Aleft( 0 right) = 2000hspace{0.5in}Aleft( 2 right) = 199.66hspace{0.5in}Aleft( {10} right) = 1999.94]

So, the maximum amount in the account will be $2000 which occurs at (t = 0) and the minimum amount in the account will be $199.66 which occurs at the 2 year mark.

In this example there are two important things to note. First, if we had included the second critical point we would have gotten an incorrect answer for the maximum amount so it’s important to be careful with which critical points to include and which to exclude.

All of the problems that we’ve worked to this point had derivatives that existed everywhere and so the only critical points that we looked at were those for which the derivative is zero. Do not get too locked into this always happening. Most of the problems that we run into will be like this, but they won’t all be like this.

Let’s work another example to make this point.

Example 5 Determine the absolute extrema for the following function and interval. [Qleft( y right) = 3y{left( {y + 4} right)^{frac{2}{3}}}hspace{0.5in}{mbox{on}}hspace{0.5in}left[ { - 5, - 1} right]] Show Solution

Again, as with all the other examples here, this function is continuous on the given interval and so we know that this can be done.

First, we’ll need the derivative and make sure you can do the simplification that we did here to make the work for finding the critical points easier.

[begin{align*}Q'left( y right) & = 3{left( {y + 4} right)^{frac{2}{3}}} + 3yleft( {frac{2}{3}} right){left( {y + 4} right)^{ - frac{1}{3}}} & = 3{left( {y + 4} right)^{frac{2}{3}}} + frac{{2y}}{{{{left( {y + 4} right)}^{frac{1}{3}}}}} & = frac{{3left( {y + 4} right) + 2y}}{{{{left( {y + 4} right)}^{frac{1}{3}}}}} & = frac{{5y + 12}}{{{{left( {y + 4} right)}^{frac{1}{3}}}}}end{align*}]

So, it looks like we’ve got two critical points.

[begin{align*}y & = - 4hspace{0.5in} & & {mbox{Because the derivative doesn't exist here.}} y & = - frac{{12}}{5} hspace{0.5in} & & {mbox{Because the derivative is zero here.}}end{align*}]

Both of these are in the interval so let’s evaluate the function at these points and the end points of the interval.

[begin{align*}Qleft( { - 4} right) & = 0 & hspace{0.5in} & & Qleft( { - frac{{12}}{5}} right) & = - 9.849 Qleft( { - 5} right) & = - 15 & hspace{0.5in} & & Qleft( { - 1} right) & = - 6.241end{align*}]

Absolute Min And Max Calc

The function has an absolute maximum of zero at (y = - 4) and the function will have an absolute minimum of -15 at (y = - 5).

So, if we had ignored or forgotten about the critical point where the derivative doesn’t exist ((y = - 4)) we would not have gotten the correct answer.

In this section we’ve seen how we can use a derivative to identify the absolute extrema of a function. This is an important application of derivatives that will arise from time to time so don’t forget about it.

Show Mobile NoticeShow All NotesHide All Notes

Section 4-3 : Minimum and Maximum Values

Many of our applications in this chapter will revolve around minimum and maximum values of a function. While we can all visualize the minimum and maximum values of a function we want to be a little more specific in our work here. In particular, we want to differentiate between two types of minimum or maximum values. The following definition gives the types of minimums and/or maximums values that we’ll be looking at.

Definition

1. We say that (fleft( x right)) has an absolute (or global) maximum at (x = c) if(fleft( x right) le fleft( c right)) for every (x) in the domain we are working on.
   
2. We say that (fleft( x right)) has a relative (or local) maximum at (x = c) if (fleft( x right) le fleft( c right)) for every (x) in some open interval around (x = c).
   
3. We say that (fleft( x right)) has an absolute (or global) minimum at (x = c) if (fleft( x right) ge fleft( c right)) for every (x) in the domain we are working on.
   
4. We say that (fleft( x right)) has a relative (or local) minimum at (x = c) if(fleft( x right) ge fleft( c right)) for every (x) in some open interval around (x = c).

Note that when we say an “open interval around(x = c)” we mean that we can find some interval (left( {a,b} right)), not including the endpoints, such that (a < c < b). Or, in other words, (c) will be contained somewhere inside the interval and will not be either of the endpoints.

Also, we will collectively call the minimum and maximum points of a function the extrema of the function. So, relative extrema will refer to the relative minimums and maximums while absolute extrema refer to the absolute minimums and maximums.

Now, let’s talk a little bit about the subtle difference between the absolute and relative in the definition above.

We will have an absolute maximum (or minimum) at (x = c) provided (fleft( c right)) is the largest (or smallest) value that the function will ever take on the domain that we are working on. Also, when we say the “domain we are working on” this simply means the range of (x)’s that we have chosen to work with for a given problem. There may be other values of (x) that we can actually plug into the function but have excluded them for some reason.

A relative maximum or minimum is slightly different. All that’s required for a point to be a relative maximum or minimum is for that point to be a maximum or minimum in some interval of (x)’s around (x = c). There may be larger or smaller values of the function at some other place, but relative to (x = c), or local to (x = c), (fleft( c right)) is larger or smaller than all the other function values that are near it.

Note as well that in order for a point to be a relative extrema we must be able to look at function values on both sides of (x = c) to see if it really is a maximum or minimum at that point. This means that relative extrema do not occur at the end points of a domain. They can only occur interior to the domain.

There is actually some debate on the preceding point. Some folks do feel that relative extrema can occur on the end points of a domain. However, in this class we will be using the definition that says that they can’t occur at the end points of a domain. This will be discussed in a little more detail at the end of the section once we have a relevant fact taken care of.

It’s usually easier to get a feel for the definitions by taking a quick look at a graph.

For the function shown in this graph we have relative maximums at (x = b) and (x = d). Both of these points are relative maximums since they are interior to the domain shown and are the largest point on the graph in some interval around the point. We also have a relative minimum at (x = c) since this point is interior to the domain and is the lowest point on the graph in an interval around it. The far-right end point, (x = e), will not be a relative minimum since it is an end point.

The function will have an absolute maximum at (x = d) and an absolute minimum at (x = a). These two points are the largest and smallest that the function will ever be. We can also notice that the absolute extrema for a function will occur at either the endpoints of the domain or at relative extrema. We will use this idea in later sections so it’s more important than it might seem at the present time.

Let’s take a quick look at some examples to make sure that we have the definitions of absolute extrema and relative extrema straight.

Example 1 Identify the absolute extrema and relative extrema for the following function. [fleft( x right) = {x^2}hspace{0.25in}{mbox{on}}hspace{0.25in}left[ { - 1,2} right]] Show Solution

Since this function is easy enough to graph let’s do that. However, we only want the graph on the interval (left[ { - 1,2} right]). Here is the graph,

Note that we used dots at the end of the graph to remind us that the graph ends at these points.

We can now identify the extrema from the graph. It looks like we’ve got a relative and absolute minimum of zero at (x = 0) and an absolute maximum of four at (x = 2). Note that (x = - 1) is not a relative maximum since it is at the end point of the interval.

This function doesn’t have any relative maximums.

As we saw in the previous example functions do not have to have relative extrema. It is completely possible for a function to not have a relative maximum and/or a relative minimum.

Example 2 Identify the absolute extrema and relative extrema for the following function. [fleft( x right) = {x^2}hspace{0.25in}{mbox{on}}hspace{0.25in}left[ { - 2,2} right]] Show Solution

Here is the graph for this function.

In this case we still have a relative and absolute minimum of zero at (x = 0). We also still have an absolute maximum of four. However, unlike the first example this will occur at two points, (x = - 2) and (x = 2).

Again, the function doesn’t have any relative maximums.

As this example has shown there can only be a single absolute maximum or absolute minimum value, but they can occur at more than one place in the domain.

Example 3 Identify the absolute extrema and relative extrema for the following function. [fleft( x right) = {x^2}] Show Solution

In this case we’ve given no domain and so the assumption is that we will take the largest possible domain. For this function that means all the real numbers. Here is the graph.

In this case the graph doesn’t stop increasing at either end and so there are no maximums of any kind for this function. No matter which point we pick on the graph there will be points both larger and smaller than it on either side so we can’t have any maximums (of any kind, relative or absolute) in a graph.

We still have a relative and absolute minimum value of zero at (x = 0).

So, some graphs can have minimums but not maximums. Likewise, a graph could have maximums but not minimums.

Example 4 Identify the absolute extrema and relative extrema for the following function. [fleft( x right) = {x^3}hspace{0.25in}{mbox{on}}hspace{0.25in}left[ { - 2,2} right]] Show Solution

Here is the graph for this function.

This function has an absolute maximum of eight at (x = 2) and an absolute minimum of negative eight at (x = - 2). This function has no relative extrema.

So, a function doesn’t have to have relative extrema as this example has shown.

Example 5 Identify the absolute extrema and relative extrema for the following function. [fleft( x right) = {x^3}] Show Solution

Again, we aren’t restricting the domain this time so here’s the graph.

In this case the function has no relative extrema and no absolute extrema.

As we’ve seen in the previous example functions don’t have to have any kind of extrema, relative or absolute.

Example 6 Identify the absolute extrema and relative extrema for the following function. [fleft( x right) = cos left( x right)] Show Solution

We’ve not restricted the domain for this function. Here is the graph.

Cosine has extrema (relative and absolute) that occur at many points. Cosine has both relative and absolute maximums of 1 at

[x = ldots - 4pi , - 2pi ,0,2pi ,4pi , ldots ]

Cosine also has both relative and absolute minimums of -1 at

[x = ldots - 3pi , - pi ,pi ,3pi , ldots ]

As this example has shown a graph can in fact have extrema occurring at a large number (infinite in this case) of points.

We’ve now worked quite a few examples and we can use these examples to see a nice fact about absolute extrema. First let’s notice that all the functions above were continuous functions. Next notice that every time we restricted the domain to a closed interval (i.e. the interval contains its end points) we got absolute maximums and absolute minimums. Finally, in only one of the three examples in which we did not restrict the domain did we get both an absolute maximum and an absolute minimum.

These observations lead us the following theorem.

Extreme Value Theorem

Suppose that (fleft( x right)) is continuous on the interval (left[ {a,b} right]) then there are two numbers (a le c,d le b) so that (fleft( c right)) is an absolute maximum for the function and (fleft( d right)) is an absolute minimum for the function.

So, if we have a continuous function on an interval (left[ {a,b} right]) then we are guaranteed to have both an absolute maximum and an absolute minimum for the function somewhere in the interval. The theorem doesn’t tell us where they will occur or if they will occur more than once, but at least it tells us that they do exist somewhere. Sometimes, all that we need to know is that they do exist.

This theorem doesn’t say anything about absolute extrema if we aren’t working on an interval. We saw examples of functions above that had both absolute extrema, one absolute extrema, and no absolute extrema when we didn’t restrict ourselves down to an interval.

The requirement that a function be continuous is also required in order for us to use the theorem. Consider the case of

[fleft( x right) = frac{1}{{{x^2}}}hspace{0.25in}{mbox{on}}hspace{0.25in}[ - 1,1]]

Here’s the graph.

This function is not continuous at (x = 0) as we move in towards zero the function is approaching infinity. So, the function does not have an absolute maximum. Note that it does have an absolute minimum however. In fact the absolute minimum occurs twice at both (x = - 1) and (x = 1).

If we changed the interval a little to say,

[fleft( x right) = frac{1}{{{x^2}}}hspace{0.25in}{mbox{on}}hspace{0.25in}left[ {frac{1}{2},1} right]]

the function would now have both absolute extrema. We may only run into problems if the interval contains the point of discontinuity. If it doesn’t then the theorem will hold.

We should also point out that just because a function is not continuous at a point that doesn’t mean that it won’t have both absolute extrema in an interval that contains that point. Below is the graph of a function that is not continuous at a point in the given interval and yet has both absolute extrema.

This graph is not continuous at (x = c), yet it does have both an absolute maximum ((x = b)) and an absolute minimum ((x = c)). Also note that, in this case one of the absolute extrema occurred at the point of discontinuity, but it doesn’t need to. The absolute minimum could just have easily been at the other end point or at some other point interior to the region. The point here is that this graph is not continuous and yet does have both absolute extrema

The point of all this is that we need to be careful to only use the Extreme Value Theorem when the conditions of the theorem are met and not misinterpret the results if the conditions aren’t met. Macos mojave download for vmware.

In order to use the Extreme Value Theorem we must have an interval that includes its endpoints, often called a closed interval, and the function must be continuous on that interval. If we don’t have a closed interval and/or the function isn’t continuous on the interval then the function may or may not have absolute extrema.

We need to discuss one final topic in this section before moving on to the first major application of the derivative that we’re going to be looking at in this chapter.

Fermat’s Theorem

If (fleft( x right)) has a relative extrema at (x = c) and (f'left( c right)) exists then (x = c) is a critical point of (fleft( x right)). In fact, it will be a critical point such that (f'left( c right) = 0).

Mac os sierra review. To see the proof of this theorem see the Proofs From Derivative Applications section of the Extras chapter.

Absolute Minimum Finder

Also note that we can say that (f'left( c right) = 0) because we are also assuming that (f'left( c right)) exists.

This theorem tells us that there is a nice relationship between relative extrema and critical points. In fact, it will allow us to get a list of all possible relative extrema. Since a relative extrema must be a critical point the list of all critical points will give us a list of all possible relative extrema.

Consider the case of (fleft( x right) = {x^2}). We saw that this function had a relative minimum at (x = 0) in several earlier examples. So according to Fermat’s theorem (x = 0) should be a critical point. The derivative of the function is,

[f'left( x right) = 2x]

Sure enough (x = 0) is a critical point.

Be careful not to misuse this theorem. It doesn’t say that a critical point will be a relative extrema. To see this, consider the following case.

[fleft( x right) = {x^3}hspace{0.25in}hspace{0.25in}f'left( x right) = 3{x^2}]

Clearly (x = 0) is a critical point. However, we saw in an earlier example this function has no relative extrema of any kind. So, critical points do not have to be relative extrema.

Also note that this theorem says nothing about absolute extrema. An absolute extrema may or may not be a critical point.

Finding Maximum And Minimum Values

Before we leave this section we need to discuss a couple of issues.

First, Fermat’s Theorem only works for critical points in which (f'left( c right) = 0). This does not, however, mean that relative extrema won’t occur at critical points where the derivative does not exist. To see this consider (fleft( x right) = left| x right|). This function clearly has a relative minimum at (x = 0) and yet in a previous section we showed in an example that (f'left( 0 right)) does not exist.

What this all means is that if we want to locate relative extrema all we really need to do is look at the critical points as those are the places where relative extrema may exist.

Absolute Minimum

Finally, recall that at that start of the section we stated that relative extrema will not exist at endpoints of the interval we are looking at. The reason for this is that if we allowed relative extrema to occur there it may well (and in fact most of the time) violate Fermat’s Theorem. There is no reason to expect end points of intervals to be critical points of any kind. Therefore, we do not allow relative extrema to exist at the endpoints of intervals.